Spin-Polarized Scanning Tunneling Microscopy

Literature Review by Dmitri Y. Petrovykh

III. Spin-Polarized Tunneling

The single most important property of ferromagnetic materials is that their electron energy bands are split into two subbands. The splitting is due to electrons' spin and it results in one of the subbands containing more electrons than the other. The two subbands are usually referred to as majority and minority subbands. Unequal population of the two subbands produces ferromagnetic materials that have non-zero magnetization without any external magnetic field.

Now let us define spin-polarization of the tunneling current P as follows:

where n "up" and "down" are densities of electrons with spins parallel and antiparallel to magnetic field, respectively. This definition of P suggests that the band splitting in ferromagnetic materials, described above, can be used to emit spin-polarized electrons. In fact, they are produced in field emission and tunneling through metal-insulator-metal junction experiments.

The simplest model for spin-dependent tunneling suggests that the spin-polarization of electrons extracted from the material is determined by the total density of states and thus can be both positive and negative. First experiments on spin-polarized field emission from well-defined facets of monocrystalline metals have been performed in late 1960s when this effect was observed for gadolinium⁸ and later for nickel⁹. Most surprising result was that the polarization turned out to be always positive and generally higher than the values predicted by the simple band-theory. Similar phenomena were observed in planar junction experiments¹⁰.

Several attempts were made to explain the results in the framework of the bulk band structure¹¹, many-body effects and spin-dependent surface potential¹². The two currently most accepted models were proposed by Slonczewski¹³ and by Stearns¹⁴ and Julliere¹⁵.

Slonczewski's theory treats electrons as independent particles, and considers two separate currents of spin-up and spin-down electrons contributing to the total tunneling current. In this approach the conductance can vary greatly in magnitude and sign depending on the wave vectors k "up" and "down" inside the ferromagnet and attenuation coefficient k in the barrier.

Model of Stearns and Julliere, regarded by some as more physical (Fig. 2), assumes that the tunneling current is proportional to the density of states of the highly-polarized itinerant d-like electrons. This theory easily explains the proportionality of spin-polarization to bulk magnetization - a very important experimental observation. However the polarization values are not well predicted for all ferromagnet-insulator-ferromagnet tunneling systems.

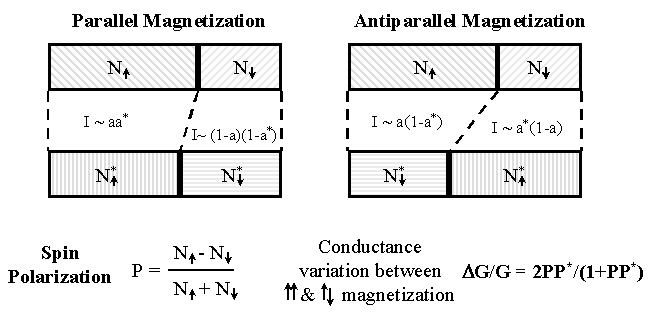
Fig. 2 Simplified version of Stearns-Julliere model of spin-polarized tunneling. N's are respective numbers spin-up, spin-down electrons in the two materials, a and a* are respective proportions of spin-up (that is aligned with the field) electrons.

At present data are too conflicting to confirm or rule out either of the models and SPSTM may make a significant contribution, providing results from well-characterized surfaces in UHV environment.

Main page: Spin-Polarized STM | Next section: SPSTM with Magnetic Tips »